Publikationen

Artikel

• Michael Quellmalz:
  The Funk-Radon transform for hyperplane sections through a common point
  Analysis and Mathematical Physics 10(38), 2020. doi:10.1007/s13324-020-00383-2 (Open Access). (pdf)

• Michael Quellmalz, Ralf Hielscher und Alfred K. Louis:
  The cone-beam transform and spherical convolution operators
  Inverse Problems 34 (2018) 105006. doi:10.1088/1361-6420/aad679
  ArXiv preprint: 1803.10515. (pdf)

• Ralf Hielscher, Daniel Potts und Michael Quellmalz:
  An SVD in Spherical Surface Wave Tomography
  In: Bernd Hofmann, Antonio Leitao and Jorge P. Zubelli (Eds.). New Trends in Parameter Identification for Mathematical Models, p. 121-144, Birkhäuser Basel, 2018. doi:10.1007/978-3-319-70824-9_7
  ArXiv preprint: 1706.05284. (pdf)

• Michael Quellmalz:
  A generalization of the Funk–Radon transform
  Inverse Problems 33 (2017) 035016. doi:10.1088/1361-6420/33/3/035016 (pdf)

• Ralf Hielscher und Michael Quellmalz:
  Reconstructing a Function on the Sphere from Its Means Along Vertical Slices
  Inverse Problems and Imaging 10(3), 2016. doi:10.3934/ipi.2016018 (pdf)

• Ralf Hielscher und Michael Quellmalz:
  Optimal Mollifiers for Spherical Deconvolution
  Inverse Problems 31 (2015) 085001. doi:10.1088/0266-5611/31/8/085001 (pdf)

Abschlussarbeiten

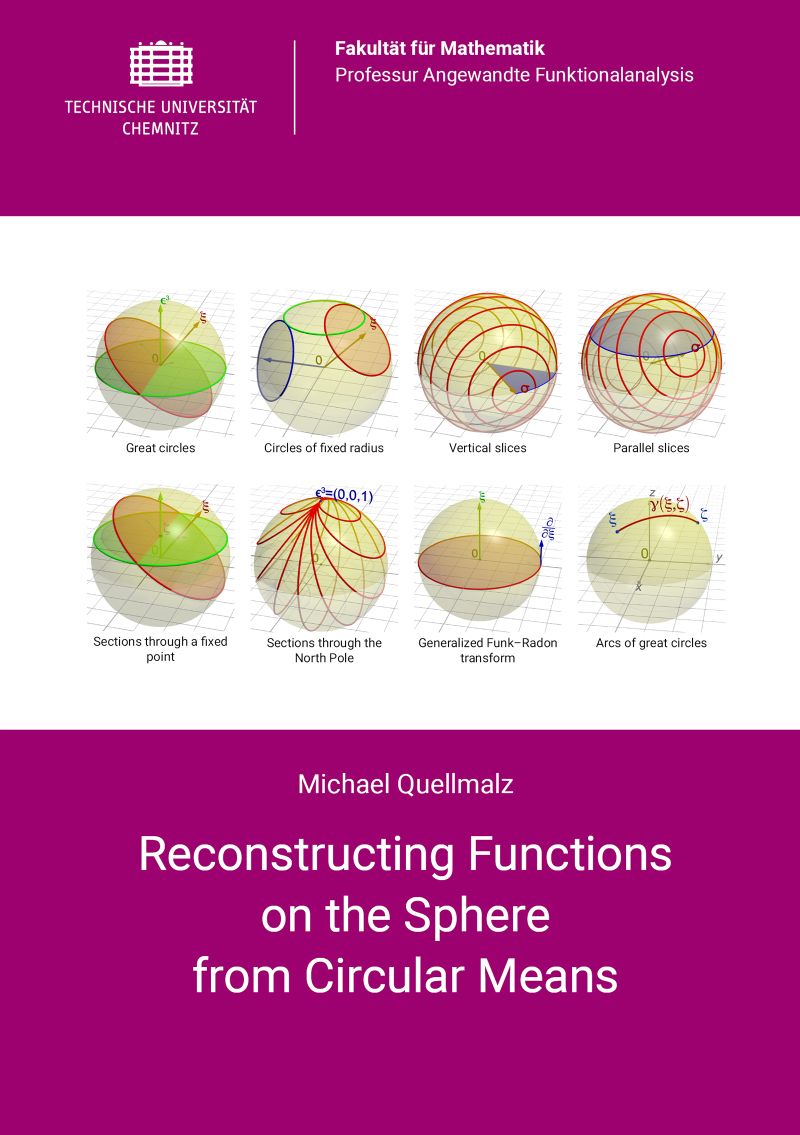
• Michael Quellmalz:
  Reconstructing Functions on the Sphere from Circular Means
  Dissertation, Universitätsverlag Chemnitz, 2019.
  ISBN 978-3-96100-116-3
  urn:nbn:de:bsz:ch1-qucosa2-384068 (pdf)

• Michael Quellmalz:
  Inversion of the Circular Average Transform on the Sphere
  Diplomarbeit, Technische Universität Chemnitz, 2014. (pdf)