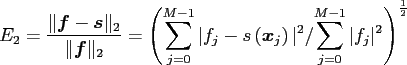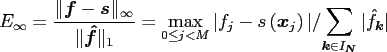Next: Computing an inverse transform
Up: Examples
Previous: Computation time vs. problem
Contents
The accuracy of the Algorithm 1, measured by
and
is shown in Figure 5.1.
Figure 5.1:
The error  (top) and
(top) and
 (bottom) with respect to
(bottom) with respect to 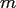 ,
from left to right
,
from left to right 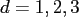 (
(
 ),
for Kaiser Bessel- (circle), Sinc- (x), B-Spline- (
),
for Kaiser Bessel- (circle), Sinc- (x), B-Spline- ( ), and Gaussian window (triangle).
), and Gaussian window (triangle).
|
|
Jens Keiner
2006-11-20